Grafy funkcí arkus sinus a arkus kosinus Arkus kosinus je jedna z cyklometrických funkcí , inverzní funkce k funkci kosinus . Obvykle se značí arccos x {\displaystyle \arccos x} a c o s x {\displaystyle \operatorname {acos\,} x} cos − 1 x {\displaystyle \cos ^{-1}x} úhel v obloukové míře (radiány) z intervalu ⟨ 0 , π ⟩ {\displaystyle \langle 0,\pi \rangle } kosinus je x {\displaystyle x}
Definice Funkce y = arccos x {\displaystyle y=\arccos x} x = cos y ( 0 ≤ y ≤ π ) {\displaystyle x=\cos y\;\left(0\leq y\leq \pi \right)} x ∈ ⟨ − 1 , 1 ⟩ {\displaystyle x\in \langle -1,1\rangle } [1]
Vlastnosti Značení: y = arccos x {\displaystyle y=\arccos x\;\;} ( resp. a c o s x , cos − 1 x ) {\displaystyle \qquad \left(\;{\mbox{resp.}}\quad \operatorname {acos\,} x,\quad \cos ^{-1}x\;\right)} [2] Definiční obor ⟨ − 1 , 1 ⟩ {\displaystyle \langle -1,1\rangle } Obor hodnot ⟨ 0 , π ⟩ {\displaystyle \langle 0,\pi \rangle } Omezenost Je omezená Monotonie Je ryze klesající ⟹ {\displaystyle \quad \Longrightarrow \quad } prostá Symetrie Není lichá sudá graf je souměrný podle středu ( x , y ) = ( 0 , π 2 ) {\displaystyle (x,y)=\left(0,{\tfrac {\pi }{2}}\right)} Periodicita Není periodická Limity lim x → 0 π 2 − arccos x x = 1 {\displaystyle \lim _{x\to 0}{\frac {{\frac {\pi }{2}}-\arccos x}{x}}=1\quad } okolí nuly je arccos x ≈ ( π 2 − x ) {\displaystyle {\mbox{arccos }}x\approx \left({\frac {\pi }{2}}-x\right)} Inverzní funkce x = cos y {\displaystyle x=\cos y} kosinus )Derivace ( arccos x ) ′ = − 1 1 − x 2 {\displaystyle (\arccos x)'=-{\frac {1}{\sqrt {1-x^{2}}}}} Integrál ∫ arccos x d x = x arccos x − 1 − x 2 + C {\displaystyle \int \arccos x\,\mathrm {d} x=x\arccos x-{\sqrt {1-x^{2}}}+C} Taylorova řada arccos x = π 2 − x − 1 2 x 3 3 − 1 ⋅ 3 2 ⋅ 4 x 5 5 − 1 ⋅ 3 ⋅ 5 2 ⋅ 4 ⋅ 6 x 7 7 − … | x | < 1 {\displaystyle \arccos x={\frac {\pi }{2}}-x-{\frac {1}{2}}{\frac {x^{3}}{3}}-{\frac {1\cdot 3}{2\cdot 4}}{\frac {x^{5}}{5}}-{\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}}{\frac {x^{7}}{7}}-\dots \qquad |x|<1\quad } Významné hodnoty x − 1 − 3 2 − 2 2 − 1 2 0 1 2 2 2 3 2 1 arccos x π 5 π 6 3 π 4 2 π 3 π 2 π 3 π 4 π 6 0 {\displaystyle {\begin{array}{c|ccc}x&-1&-{\frac {\sqrt {3}}{2}}&-{\frac {\sqrt {2}}{2}}&-{\frac {1}{2}}&0&{\frac {1}{2}}&{\frac {\sqrt {2}}{2}}&{\frac {\sqrt {3}}{2}}&1\\\hline \arccos x&\pi &{\frac {5\pi }{6}}&{\frac {3\pi }{4}}&{\frac {2\pi }{3}}&{\frac {\pi }{2}}&{\frac {\pi }{3}}&{\frac {\pi }{4}}&{\frac {\pi }{6}}&0\end{array}}}
Vzorce arcsin x + arccos x = π 2 arccos x + arccos ( − x ) = π arccos x + arccos y = { arccos ( x y − 1 − x 2 1 − y 2 ) , x + y ≥ 0 2 π − arccos ( x y − 1 − x 2 1 − y 2 ) , x + y < 0 arccos x − arccos y = { − arccos ( x y + 1 − x 2 1 − y 2 ) , x ≥ y arccos ( x y + 1 − x 2 1 − y 2 ) , x < y {\displaystyle {\begin{array}{lcll}\arcsin x+\arccos x&=&{\frac {\pi }{2}}\\\arccos x+\arccos(-x)&=&\pi \\\arccos x+\arccos y&=&\left\{{\begin{array}{rl}\arccos \left(xy-{\sqrt {1-x^{2}}}{\sqrt {1-y^{2}}}\right),&x+y\geq 0\\2\pi -\arccos \left(xy-{\sqrt {1-x^{2}}}{\sqrt {1-y^{2}}}\right),&x+y<0\end{array}}\right.\\\arccos x-\arccos y&=&\left\{{\begin{array}{rl}-\arccos \left(xy+{\sqrt {1-x^{2}}}{\sqrt {1-y^{2}}}\right),&x\geq y\\\arccos \left(xy+{\sqrt {1-x^{2}}}{\sqrt {1-y^{2}}}\right),&x<y\end{array}}\right.\\\end{array}}} arccos ( cos x ) = x , 0 ≤ x ≤ π cos ( arccos x ) = x {\displaystyle {\begin{array}{lcll}\arccos(\cos x)&=&x,&0\leq x\leq \pi \\\cos(\arccos x)&=&x\end{array}}}
arccos x = ∫ x 1 d t 1 − t 2 , 0 < x < 1 {\displaystyle \arccos x=\int _{x}^{1}{\frac {\mathrm {d} t}{\sqrt {1-t^{2}}}},\quad 0<x<1} arccos x = π 2 − x 1 − x 2 1 − 1 ⋅ 2 ⋅ x 2 3 − 1 ⋅ 2 ⋅ x 2 5 − 3 ⋅ 4 ⋅ x 2 7 − 3 ⋅ 4 ⋅ x 2 9 − 5 ⋅ 6 ⋅ x 2 11 − … , | x | < 1 {\displaystyle \arccos x={\frac {\pi }{2}}-{\frac {x\,{\sqrt {1-x^{2}}}}{1-\displaystyle {\frac {1\cdot 2\cdot x^{2}}{3-\displaystyle {\frac {1\cdot 2\cdot x^{2}}{5-\displaystyle {\frac {3\cdot 4\cdot x^{2}}{7-\displaystyle {\frac {3\cdot 4\cdot x^{2}}{9-\displaystyle {\frac {5\cdot 6\cdot x^{2}}{11-\dots }}}}}}}}}}}},\quad |x|<1} Příklad použití Mějme goniometrickou rovnici : [3]
2 cos x = 1 cos x = 1 2 x = arccos 1 2 x = π 3 {\displaystyle {\begin{array}{rcl}2\cos x&=&1\\\hline \cos x&=&{\frac {1}{2}}\\x&=&\arccos {\frac {1}{2}}\\\hline x&=&{\frac {\pi }{3}}\end{array}}} S ohledem na periodicitu funkce cos x {\displaystyle \cos x} jsou řešením původní rovnice také hodnoty:
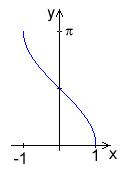
… , − 11 π 3 , − 5 π 3 , π 3 , 7 π 3 , 13 π 3 , … tj. x k = π 3 + 2 k π , k ∈ Z … , − 13 π 3 , − 7 π 3 , − π 3 , 5 π 3 , 11 π 3 , … tj. x k = − π 3 + 2 k π , k ∈ Z {\displaystyle {\begin{array}{rrrrrrrlr}\dots ,&{\color {OliveGreen}{\frac {-11\pi }{3}}},&{\color {OliveGreen}{\frac {-5\pi }{3}}},&\mathbf {\frac {\boldsymbol {\pi }}{3}} ,&{\color {OliveGreen}{\frac {7\pi }{3}}},&{\color {OliveGreen}{\frac {13\pi }{3}}},&\dots &\qquad {\mbox{tj.}}\quad \color {OliveGreen}{x_{k}=}&\color {OliveGreen}{{\frac {\pi }{3}}+2k\pi },\;k\in \mathbb {Z} \\\dots ,&{\color {BrickRed}{\frac {-13\pi }{3}}},&{\color {BrickRed}{\frac {-7\pi }{3}}},&{\color {BrickRed}{\frac {-\pi }{3}}},&{\color {BrickRed}{\frac {5\pi }{3}}},&{\color {BrickRed}{\frac {11\pi }{3}}},&\dots &\qquad {\mbox{tj.}}\quad \color {BrickRed}{x_{k}=}&\color {BrickRed}{-{\frac {\pi }{3}}+2k\pi },\;k\in \mathbb {Z} \end{array}}} Graf Vznikne překlopením grafu funkce y = cos ( x ) {\displaystyle y=\cos(x)} kvadrantu . Graf funkce arkus kosinus Odkazy Reference ↑ KAREL REKTORYS A SPOLUPRACOVNÍCI. Přehled užité matematiky. 7. vyd. Praha: Prometheus, 2000. ISBN 8071961795 ↑ WolframAlpha: arccos x ↑ WolframAlpha: 2cos(x)=1 Externí odkazy Obrázky, zvuky či videa k tématu arkus kosinus Wikimedia Commons BARTSCH, Hans-Jochen. Matematické vzorce. 3., rev. vyd. Přeložil Zdeněk TICHÝ. Praha: Mladá fronta, 1996. ISBN 80-2040607-7 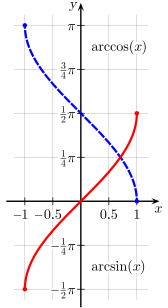
 Obrázky, zvuky či videa k tématu arkus kosinus na Wikimedia Commons
Obrázky, zvuky či videa k tématu arkus kosinus na Wikimedia Commons 
